From Knots to Fluids to Extra Dimensions, Experts Weigh in on Mathematical Challenges
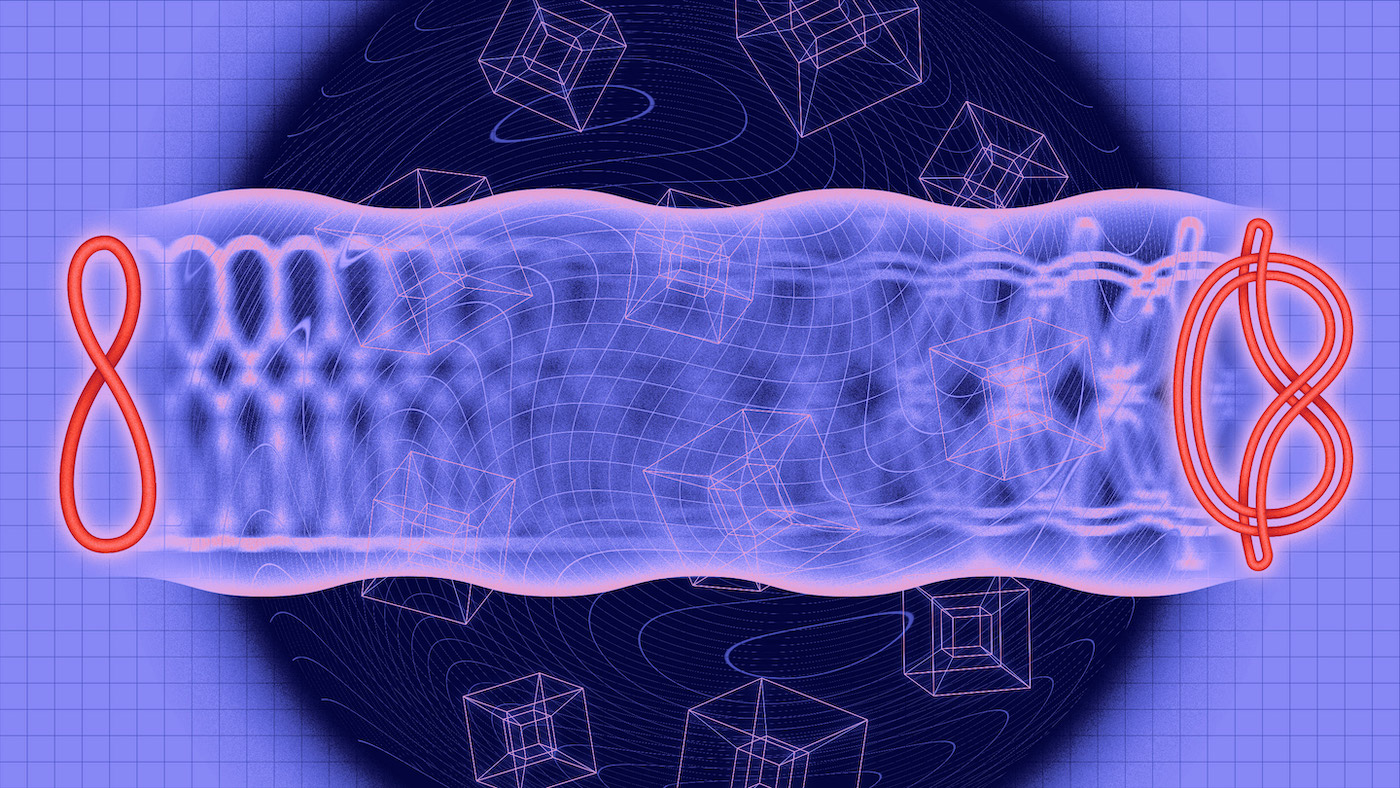
Two knots are concordant if they can be connected with a type of four-dimensional cylinder. Image by Kristina Armitage/Quanta Magazine.
Several University of Texas at Austin mathematics faculty, including an incoming assistant professor, have seen their work spotlighted in the science publication Quanta in recent weeks.
Distinct in 3D and 4D
Maggie Miller, a UT alumna, current Stanford University postdoc and soon-to-be faculty member at UT Austin, was featured in the magazine's article, "Surfaces So Different Even a Fourth Dimension Can't Make Them the Same." Miller co-authored a paper that solved a question that has bedeviled mathematicians for decades: understanding aspects of Seifert surfaces — two-dimensional surfaces whose boundary is a knot — and specifically whether there are any pairs of Seifert surfaces from the same knot that are distinct from one another in three dimensions as well as four.
"It's a common thing in topology that when you have something interesting and add an extra dimension, it might not be interesting anymore," Miller told Quanta.
The new work identifies the first pair of Seifert surfaces that are as provably distinct from each other in 4D as in 3D. The work also goes a step further by identifying additional pairs of Seifert surfaces that become alike in some ways but not in others.
Knot ranking
Mathematics professor Cameron Gordon was featured in another recent Quanta article, "How Complex Is a Knot? New Proof Reveals Ranking System That Works." As noted in the article:
Back in 1981, Cameron Gordon introduced a new way to relate two knots — mathematical constructs modeled after the knots that appear in a single thread or string. In his paper, he conjectured that this new relationship could be used to arrange groups of knots according to how complicated they are.
This winter, Ian Agol, a mathematician at the University of California, Berkeley, posted a six-page paper that proved Gordon's conjecture, giving mathematicians a new way to order knots by complexity.
"You throw [two knots] down on the table and they may look completely different, right?" Gordon told the magazine. "But if you can move one around so that it looks exactly like the other, then you say the knots are the same."
Gordon called the recent work that built upon his own a "beautifully short, beautifully elegant proof."
Fluid answers
Mathematics professor Misha Vishik was featured in the article, "Mathematicians Coax Fluid Equations Into Nonphysical Solutions," which referenced two 2018 papers he authored and how it relates to newer work mathematicians are doing to answer questions about Navier-Stokes equations, a set of equations used to describe the motion of liquids. According to the article:
Fluids are inherently difficult to describe, as their constituent molecules don't move as one. To account for this, the Navier-Stokes equations describe a fluid using "velocity fields" that specify a speed and direction for each point in 3D space. The equations describe how a starting velocity field evolves over time. The big question that mathematicians want to answer: Will the Navier-Stokes equations always work, for any starting velocity field into the arbitrarily distant future?
To answer that requires knowing whether there is "only a single solution to the Navier-Stokes equations, no matter the initial conditions." Using Vishik's research, mathematicians recently proved that non-uniqueness also occurs in three-dimensional equations.
While the most sought-after answers are about the Navier-Stokes equations in three-dimensional space, two-dimensional versions of the equations also exist. Vishik had proved that non-uniqueness occurs in a modified version of these 2D equations. … [The team behind the new work] realized they could use Vishik's vortex as the foundation for two distinct solutions in three dimensions as well.



